On instructing cells to grow into differentiated bodies. This notebook has been resurrected from the trash bin years after I deleted it because of my great enjoyment of Mordvintsev et al. (2020) on differentiable cellular automata.
1 Differentiable Cellular Automata
See learning automata.
2 Actual Biologically-Plausible Morphogenesis
I know nothing of that.
3 Abstracted Morphogenesis, mathetmatical tools for
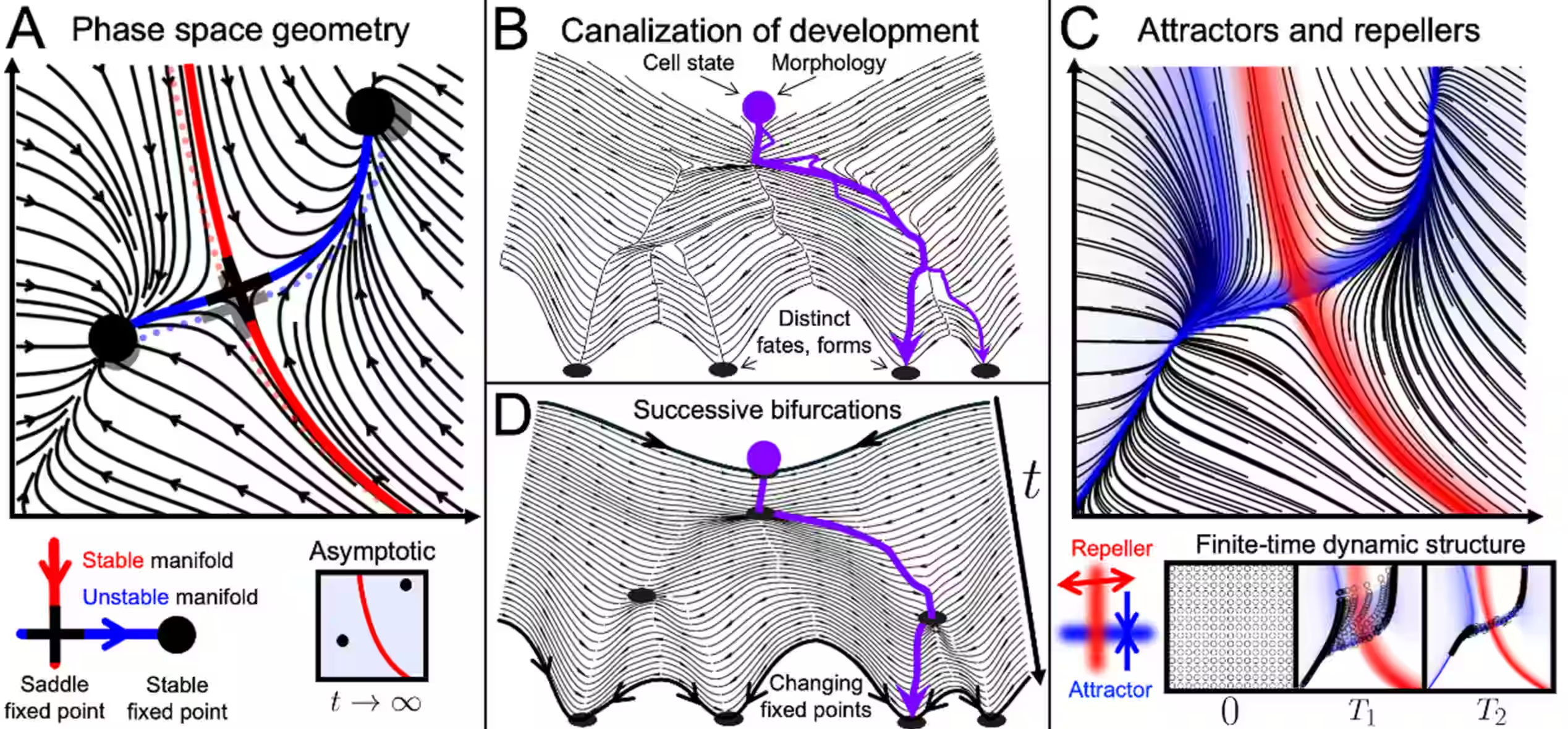
Plum and Serra (2025) looks interesting:
Developmental biology has long drawn on dynamical systems to understand the diverging fates and the emerging form of the developing embryo. Cell differentiation and morphogenesis unfold in high-dimensional geneexpression spaces and position spaces. Yet, their stable and reproducible outcomes suggest low-dimensional geometric structures—e.g., fixed points, manifolds, and dynamic attracting and repelling structures—that organize cell trajectories in both spaces. This review surveys the history and recent advances in dynamical systems frameworks for development. We focus on techniques for extracting the organizing geometric structures of cell fate decisions and morphogenetic movements from experiments, as well as their interconnections. This unifying, dynamical systems perspective aids in rationalizing increasingly complex experimental datasets, facilitating principled dimensionality reduction and an integrated understanding of development, bridging typically distinct domains.